Cyril Touzé

Recherche

Navigation

• Nonlinear Normal Modes (NNMs)
• Plates and shells
• Gongs and cymbals
• Sound Synthesis
• Cymbals
• Wave turbulence

Sound synthesis of cymbals including thickness and shape variations

Web Companion page for the paper :

Nonlinear vibrations of thin plates with variable thickness : application to sound synthesis of cymbals,

by Quoc Bao Nguyen and Cyril Touzé,
Journal of the Acoustical Society of America, vol. 145(2), 977-988, 2019.
[JASA]

This web-page is the companion page of the paper published in the Journal of the Acoustical Society of America (JASA), vol. 145(2), 977-988, 2019. The sound files associated to the paper are here given. They are produced from the modal code developed for that purpose (see paper) and more generally inserted into VK-Gong, the free software developed at the lab for nonlinear vibrations of thin plates and sound synthesis of gongs and cymbals. The WAV files are obtained from the velocity of a single point of the plate. All the simulations have been realised with N=800 modes, and a sampling rate at 100 kHz.

The main outcome of the paper is to develop a complete model for cymbal vibrations including the taper effect (thickness variation along the radius) together with the bow effect (shape variation), in order to confer the cymbal the ability to play two different tones when being hit strongly on the edge (crash sound) or on the bell (distinct tone to mark rhythm).

Decreasing the thickness at edge

a circular plate of radius 0.2 m is selected. A decreasing thickness along the radius is arranged as follows:
-the thickness is constant for r in [0, 0.05], with value 1 mm
-then the thickness decreases linearly down to the edge,
with a final thickness value h_e.
Three values of h_e are selected :
-h_e = 1 mm (i.e. plate with uniform thickness)
-h_e = 0.7 mm
-h_e = 0.4 mm

The plate is excited with a strike of amplitude 90 N. The figure below shows the displacement of one point and the corresponding spectrograms.
The associated sound files are:

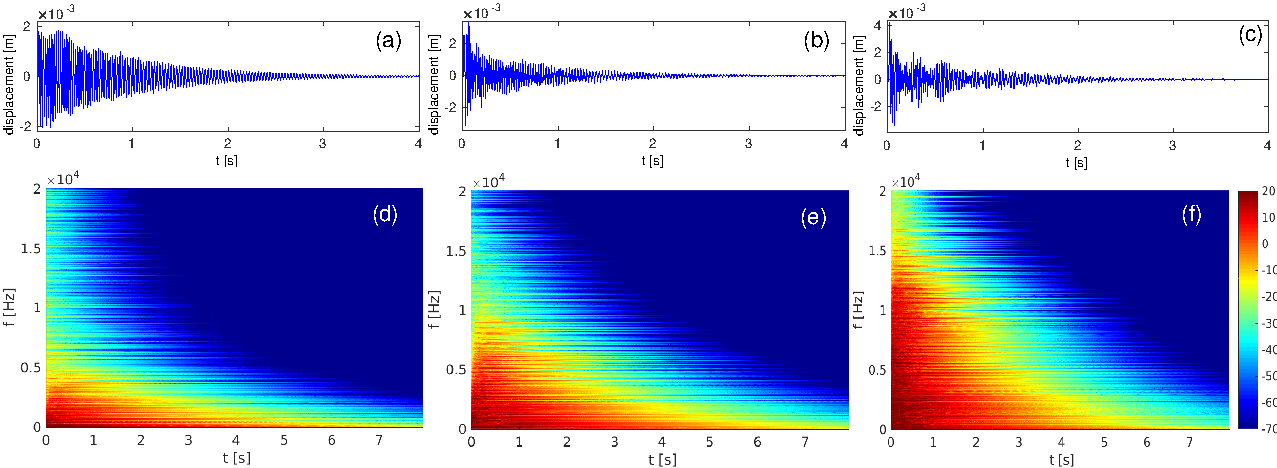
• Case 1 : plate with uniform thickness h=1mm
  
• Case 2 : plate with decreasing thickness down to h_e = 0.7 mm
  
• Case 3 : plate with decreasing thickness down to h_e = 0.4 mm
  
• Case 4 : in order to draw out a more complete comparison, the sound file generated by selecting the case of a plate with uniform and small thickness h=0.4 mm is selected here
  
  
  

The video below shows the displacement field of the cymbal with uniform thickness (on the left) compared to that of the cymbal with a linearly decreasing thickness down to h_e=0.4 mm at the edge. The amplitude of the strike has been set to 150 N. This movie is the animated version of Fig. 3 in the paper.

A complete model combining shape imperfection and variable thickness

A complete model is developed to study the combined effects of a shape imperfection and a tapered thickness. The shape imperfection is selected has shown below, following a measurement realized on a real crash cymbal.


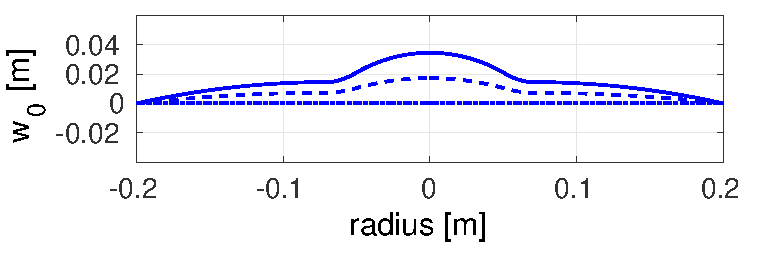
The shape is parameterized by the height at center H_c, selected either at H_c=1.7cm or H_c = 3.4 cm.
The figure below shows the spectrograms of
-cymbal with uniform thickness h = 1 mm, shape variation only with height at centre H_c = 3.4 cm.
-cymbal with the same shape variation, H_c = 3.4 cm, and linear decrease of the thickness down to h_e = 0.4 mm.
Amplitude of the strike is 90 N.


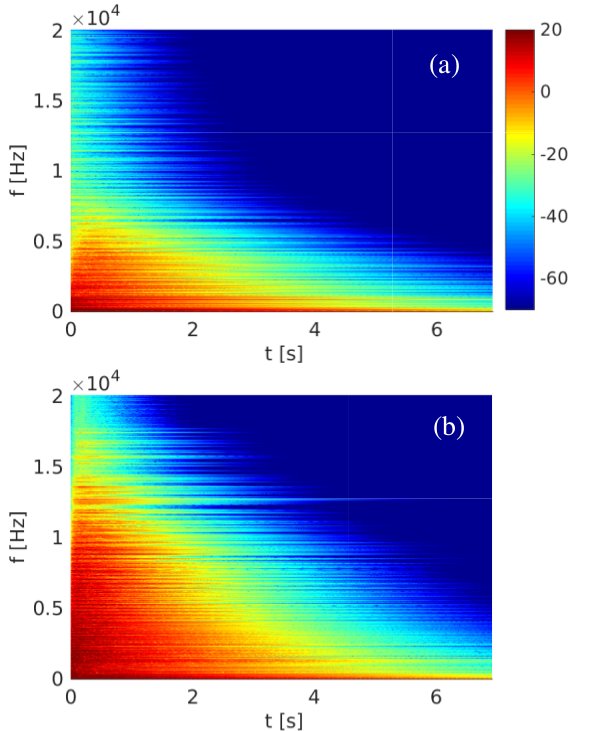
The corresponding sound files are:

• Case 1 : plate with uniform thickness h=1mm, shape variation only, H_c = 3.4 cm
  
• Case 2 : shape variation with H_c = 3.4 cm and decreasing thickness down to h_e = 0.4 mm
  
  
  

The combined effects of both shape imperfection and taper is to rigidify the center and soften the edge by playing both on the thickness variation and shape imperfection, which also has the effect of increasing the stiffness of the structure by adding curvature. Having only thickness variation without shape imperfection results in a cymbal which is not stiff enough and shows too important pitch glides. On the other hand, having only shape imperfection results in a too stiff cymbal which is difficult to drive to the strongly nonlinear regime and typical crash sounds when hit at edge. Both variations allows for a good compromise between explosive sound without a too prominent pitch glide. This is exemplified with the three following sounds, corresponding to Fig. 8 of the paper, with a vigorous strike at edge of amplitude 150 N.

• Case 1 : only thickness variation down to h_e = 0.4 mm
  
• Case 2 : only shape variation with H_c = 3.4 cm
  
• Case 3 : shape and thickness variations with h_e=0.4 mm and H_c = 3.4 cm
  
  
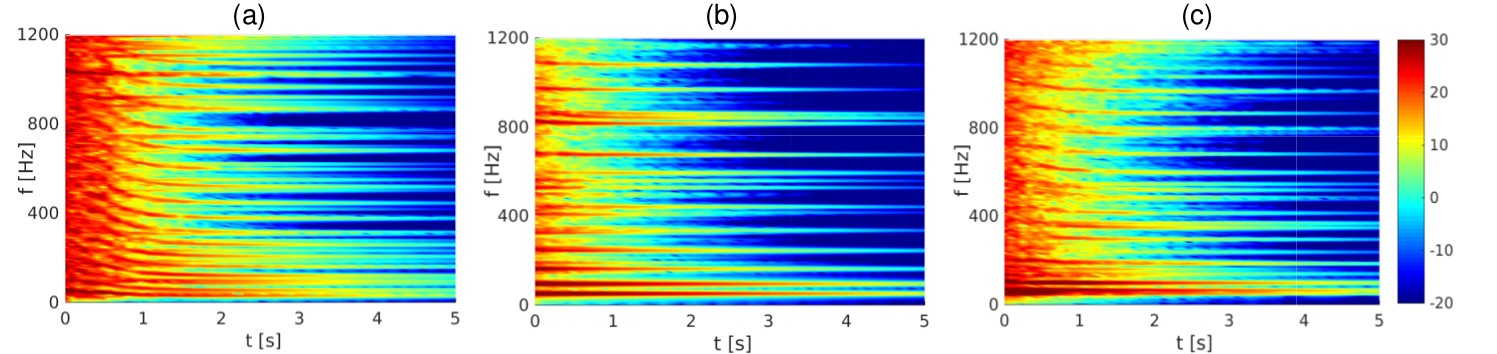
  The figure below reproduces Fig. 8 of the paper and shows clearly the different pitch glide with spectrograms restricted to the low-frequency area and limited dB range (50 dB).
  
  

The combination of both taper and shape imperfection is also crucial in order to assess the ability of a cymbal to play two kinds of very different sounds:
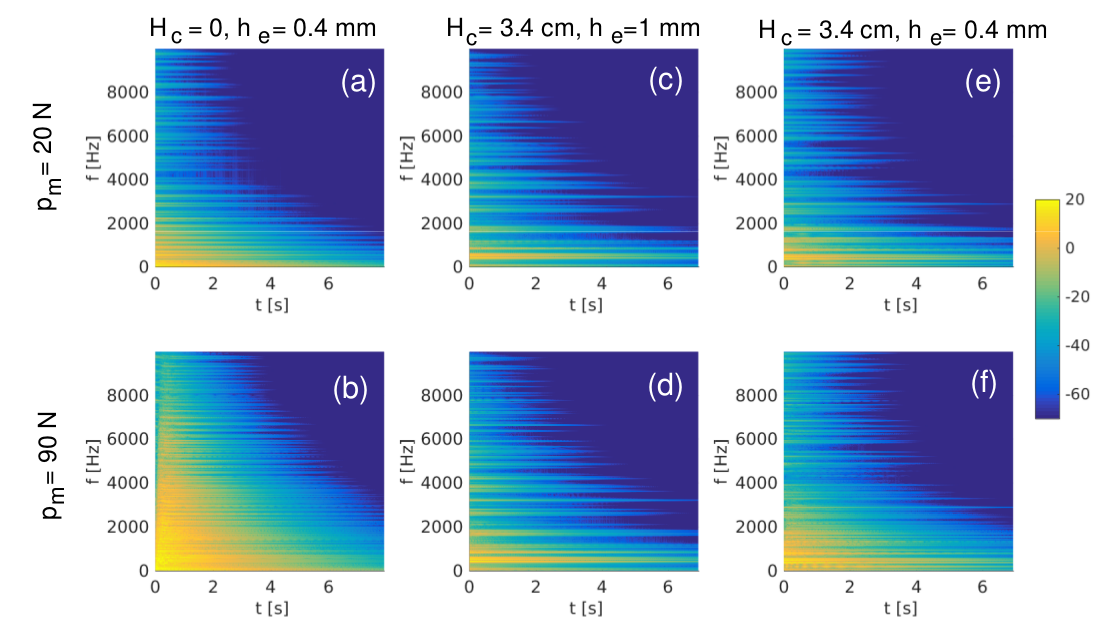
-a clear tone with a dominant pitch when hit near the centre, in the region known as the bell.
-a non-tonal, bright glittering sound is awaited when strongly striking crash and splash cymbals at the edge.
The sounds given below, corresponding to Fig. 9 of the paper, exemplifies this by hearing at the sounds generated when a cymbal is struck at center. The following cases are to be heard:

• Case (a) : only thickness variation down to h_e = 0.4 mm, strike at center with amplitude 20 N
  
• Case (b) : same as (a) with a more vigorous strike at 90 N
  
• Case (c) : only shape variation with H_c = 3.4 cm, strike at center with amplitude 20 N
  
• Case (d) : same as (c) with a more vigorous strike at 90 N
  
• Case (e) : thickness and shape variation with h_e=0.4mm and H_c = 3.4 cm, strike at center with amplitude 20 N
  
• Case (f) : same as (e) with a more vigorous strike at 90 N
  
  
  The figure below reproduces Fig. 9 of the paper and showing the spectrograms corresponding to the sounds of cases (a) to (f) explained above
  
  
  
  
  
  
  
  
  

  Page created on february 27th, 2019